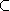
 /n
/n , of
the same number of elements, and asks you to transform
E into F by successive additions and multiplications by
elements of
, of
the same number of elements, and asks you to transform
E into F by successive additions and multiplications by
elements of  /n
/n . And you will be scored according to the number
of steps you make.
. And you will be scored according to the number
of steps you make.
 /n
/n , represented by k integers
between 0 and n-1. For any element t
, represented by k integers
between 0 and n-1. For any element t 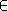
 /n
/n , the addition
or multiplication by t transforms E into another set
Et
, the addition
or multiplication by t transforms E into another set
Et 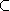
 /n
/n .
.
Thus the exercise generates two sets E,F 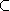
 /n
/n , of
the same number of elements, and asks you to transform
E into F by successive additions and multiplications by
elements of
, of
the same number of elements, and asks you to transform
E into F by successive additions and multiplications by
elements of  /n
/n . And you will be scored according to the number
of steps you make.
. And you will be scored according to the number
of steps you make.
Choose the level of difficulty: 1 2 3 4 5 6 7 8 9
You can also configure the exercise by
a detailed menu.
The most recent version
Please take note that WIMS pages are interactively generated; they are not ordinary HTML files. They must be used interactively ONLINE. It is useless for you to gather them through a robot program.